---

Investigate the different effects a and b have on the parametric equations when .

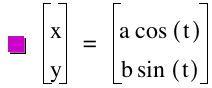, where a = b.

Then you'll have a graph like this.

It appears to be a circle with origina as a center and radius 1.

What if I increase a and b? Make sure a = b. With this example, a = b = 2.

Then it is a circle with the origin as the center and radius is 2.

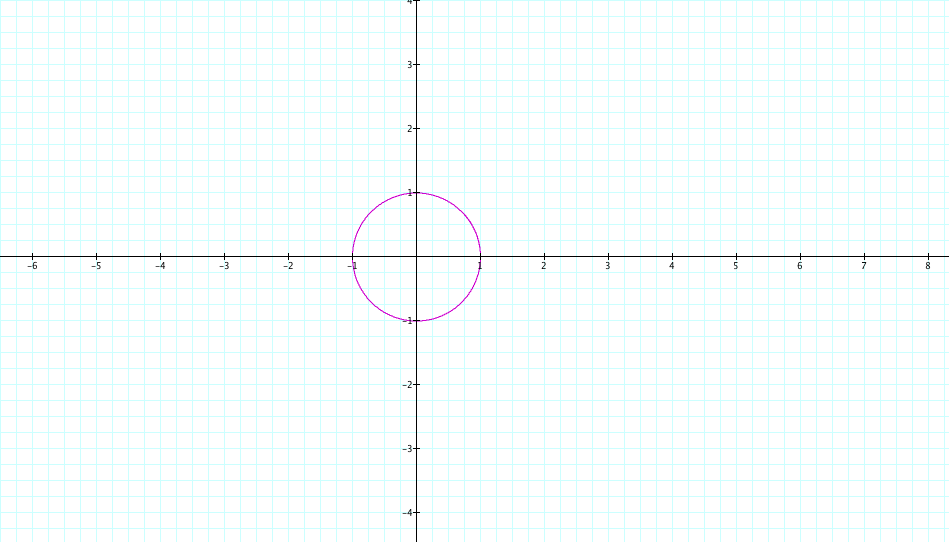
Let a = b = 6.

Then it is a circle with the origin as the center and the radius is 2.

This time, I made a and b as a negative number. a = b =-4

It still appears as a circle with the origin as the center and the radius is 4.

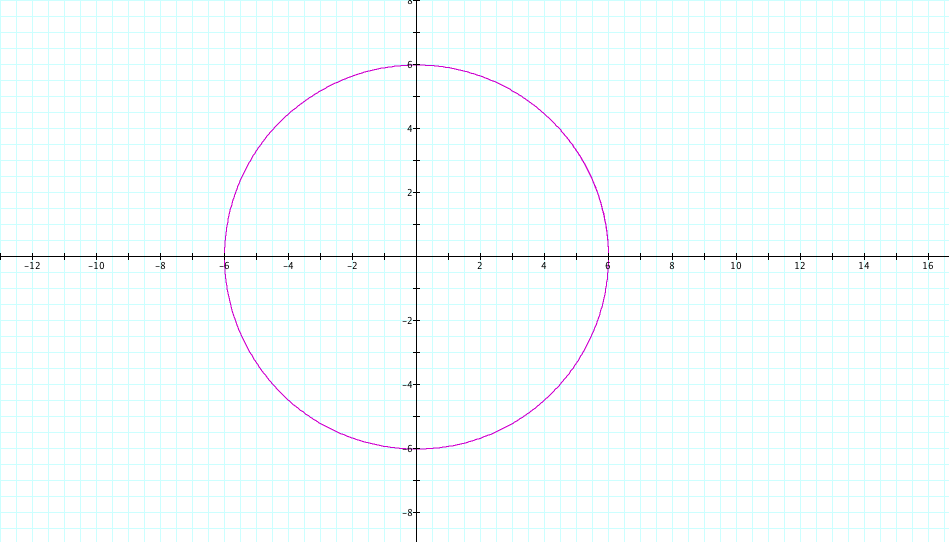
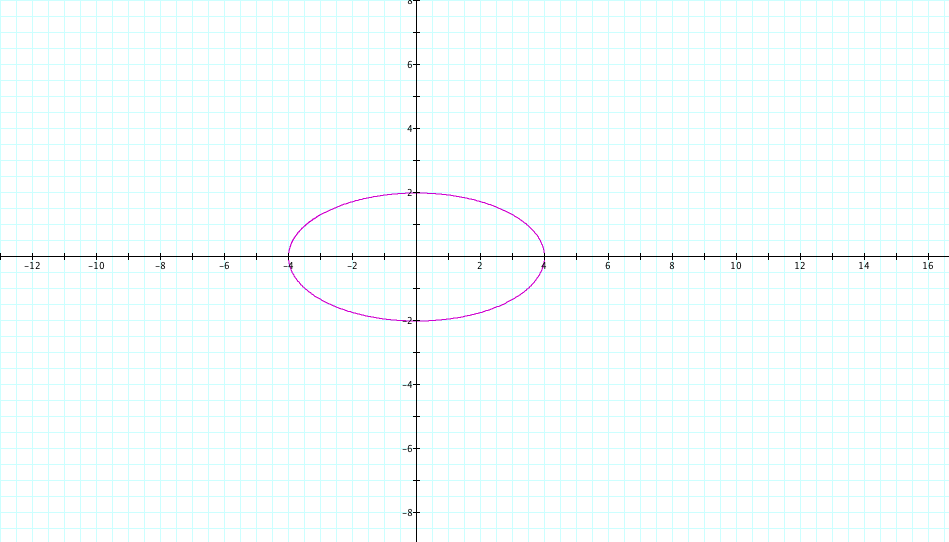
Now, let's make a > b. a = 4 and b = 2.

Then it appears to be an ellipse where the origin is the center. However, it appears longer on the x-axis than the y-axis. Probably because a > b. Let's look at more examples to make sure if this is the case.

a = 7 and b = 3

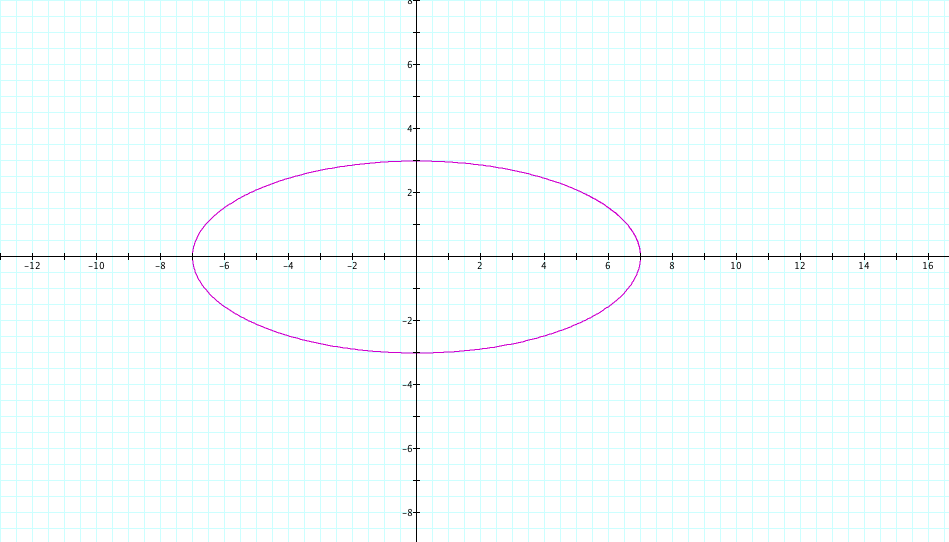
a = 6 and b = 1

What happens if a > b mathematically? That means a is a positive number where b is a negative number, yet b is greater when you apply the absolute value to both values.

a = 5 and b= -7

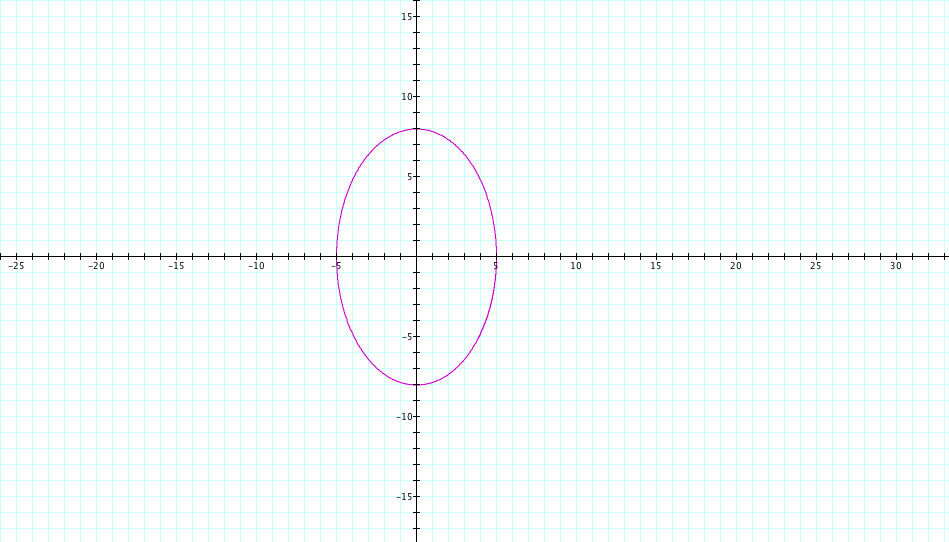
The ellipse looks different from the previous examples. Instead of stretching out further on the x-axis, it stretches more on the y-axis. So, when a > b, it means when you apply the absolute value to both values, you compare the numbers by their magnitute.

From what I've observed, I'm going to assume when a < b, then the ellipse will stretch further on the y-axis than the x-axis since b is greater than a.

a = 1 and b = 4	a = 3 and b = 5	a = 5 and b = 8

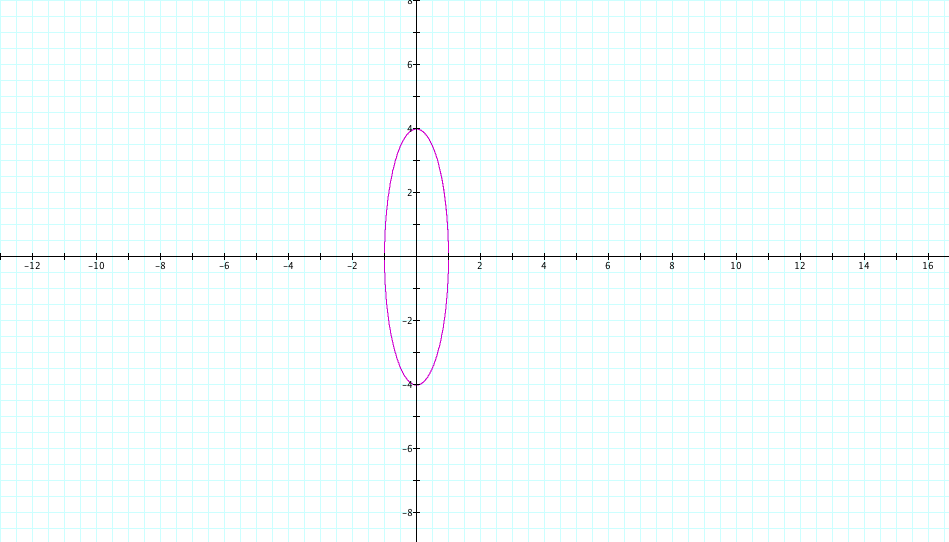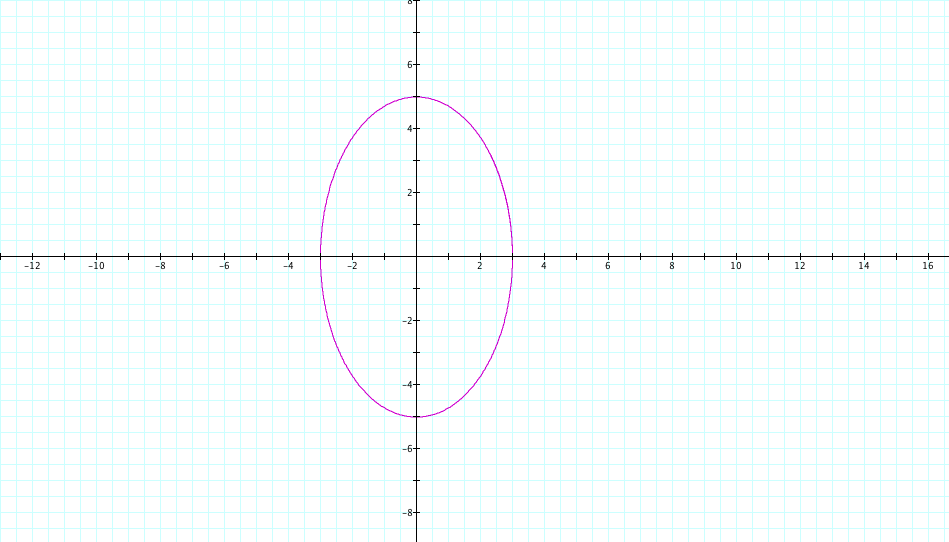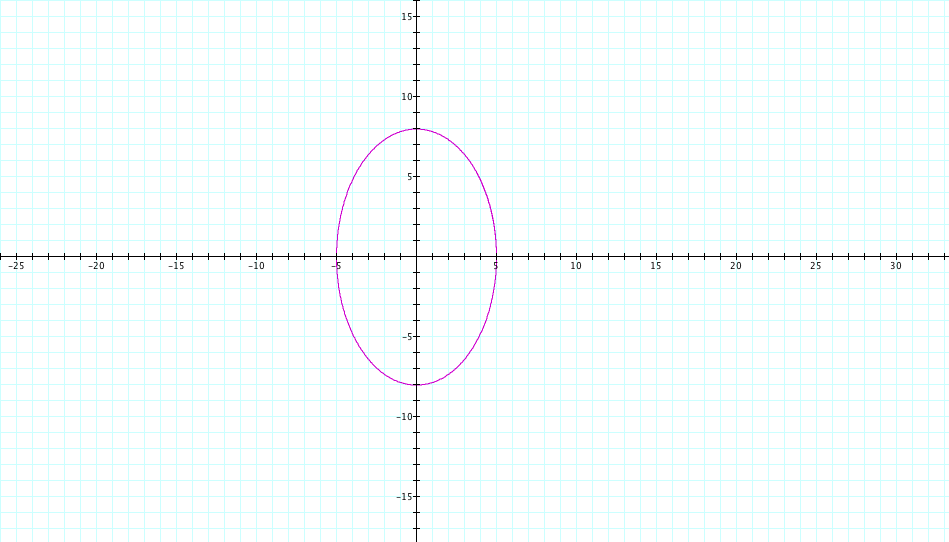Also, I'm curious what happens if a < b mathematically as well? a = -4 and b = 2

Since |a| > |b| even though a < b mathematically, the ellipse is stretched more on the x-axis than the y-axis.

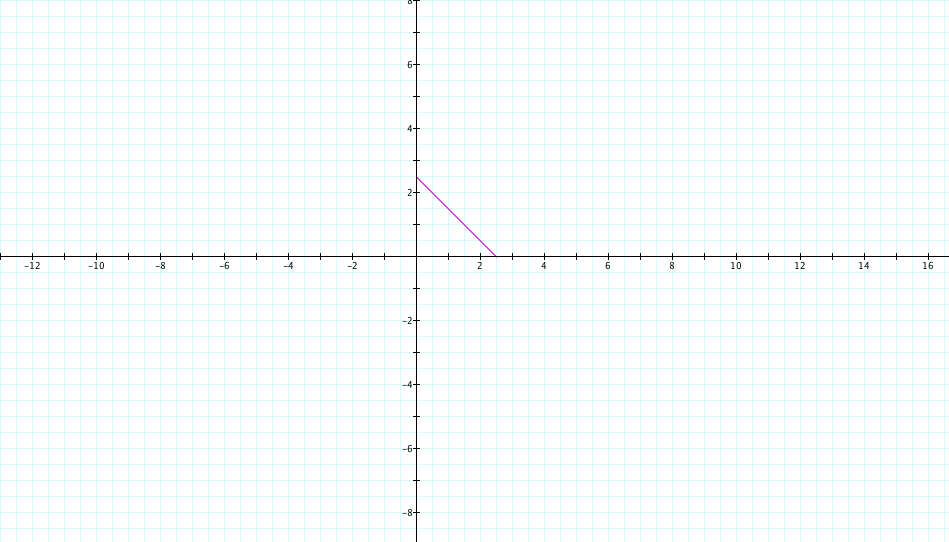
Now let's investigate when . First, let's investigate when a = b.

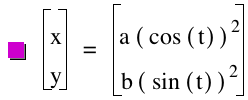
It appears to be a straight line that connects (1, 0) and (0, 1).

a = b= 2.5	a = b = 3	a = b = 6

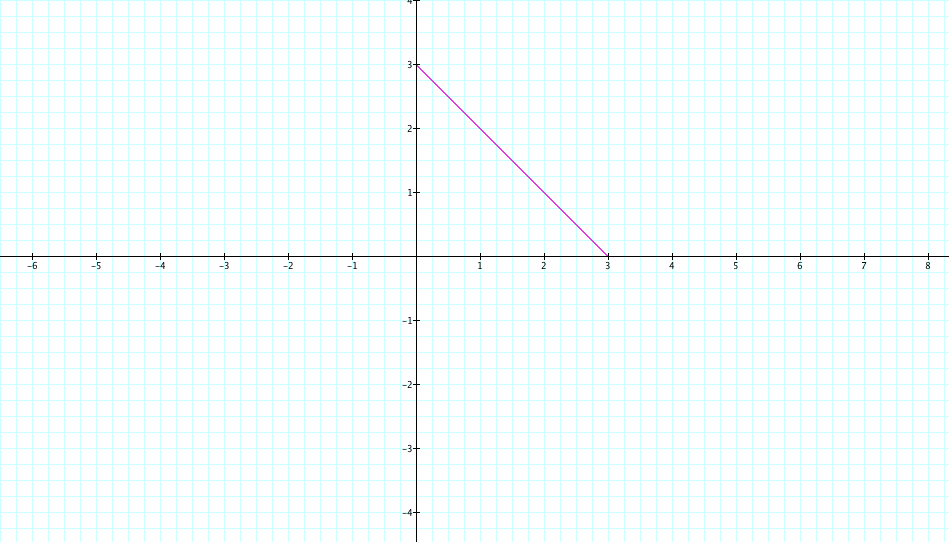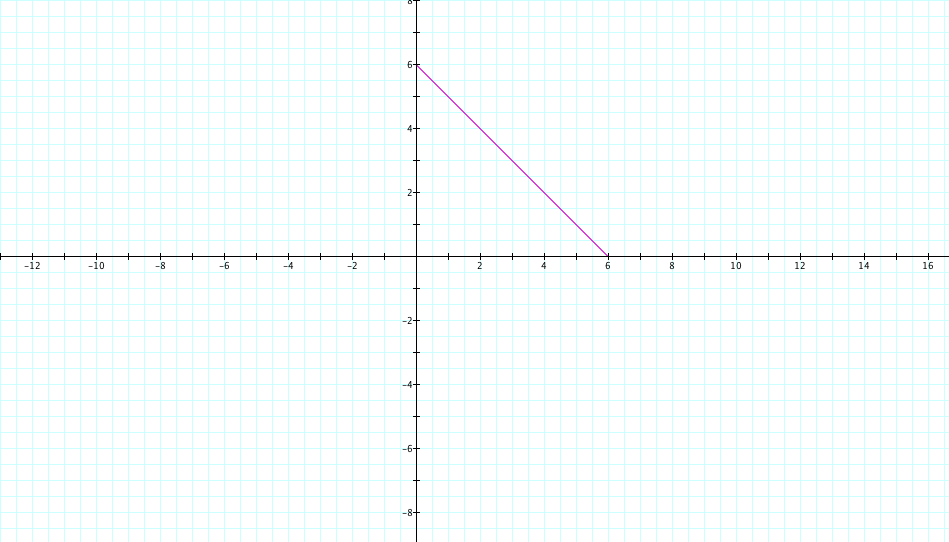When the degree was raised to the second power, the circle(ellipse) has changed into a straight line. (a or (b), 0) will be the x-intercept and (0, a or (b)) will be the y-intercept.

What if a > b?

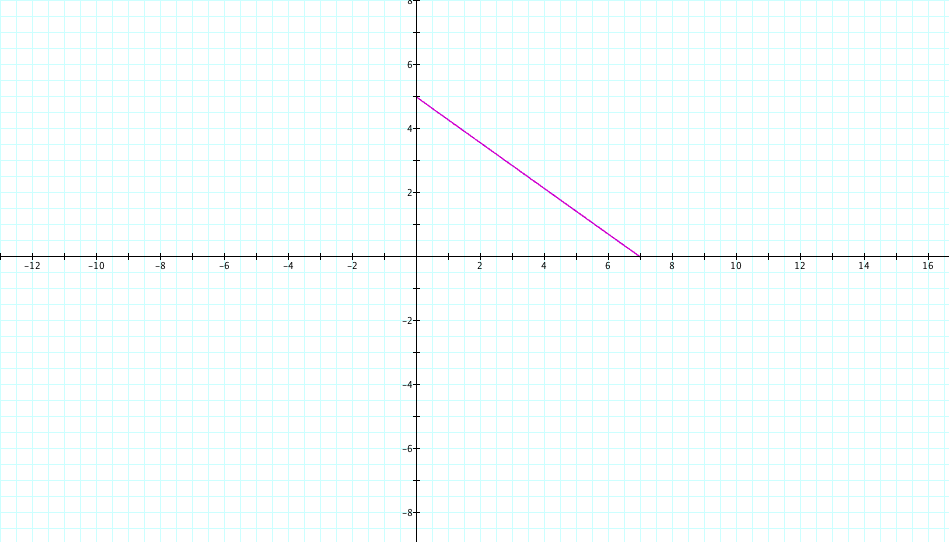
a = 2 and b = 1	a = 7 and b = 5	a = 15 and b = 12

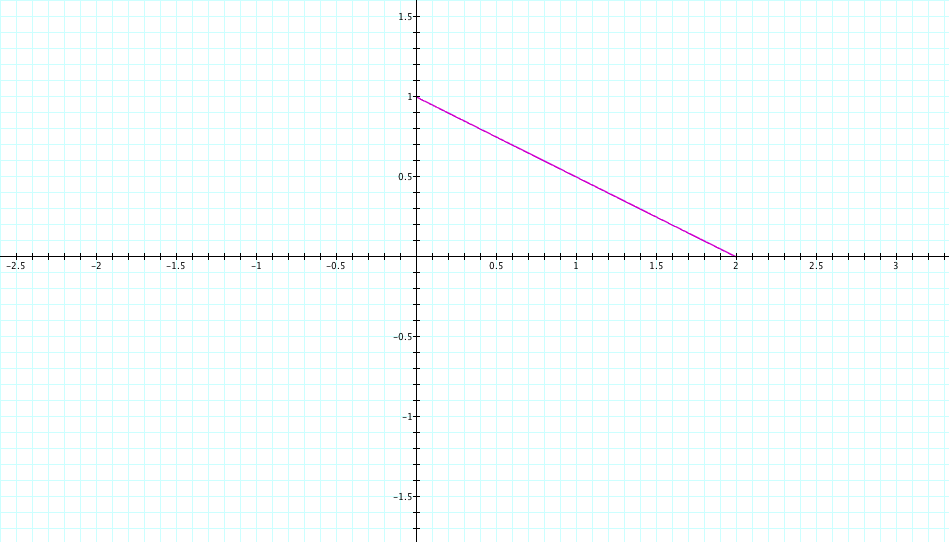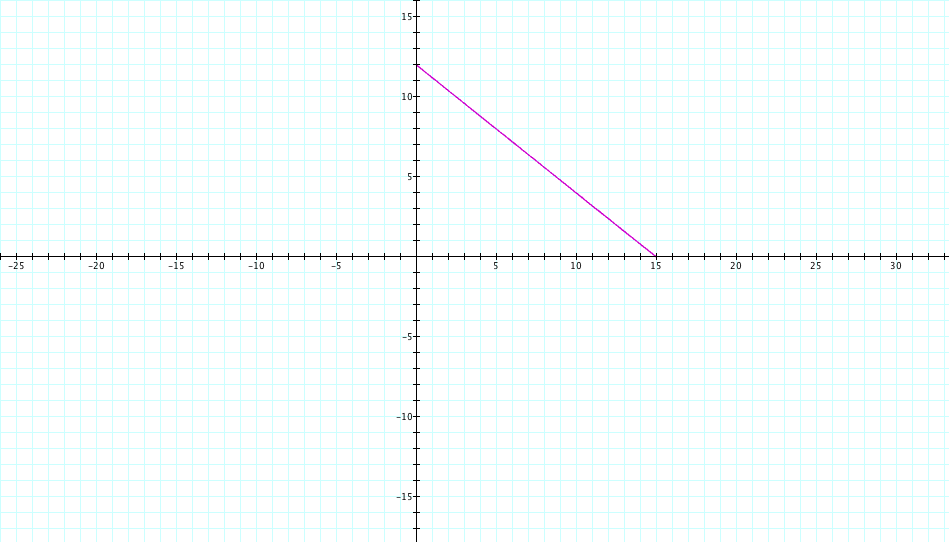Since a > b, the line extends longer on the x-axis than the y-axis. This time the x-intercept is (a, 0) and the y-intercept is (0, b).

Once again, I want to know what happens if a > b, but |a| < |b|?

a = 4 and b = -8

Even though a > b, since |a| < |b| the line extends further on the y-axis. Unlike the parametric equation in first degree, the negative value changes the direction of the graph. The x-intercept is (4, 0), but the y-intercept is (0, -8).

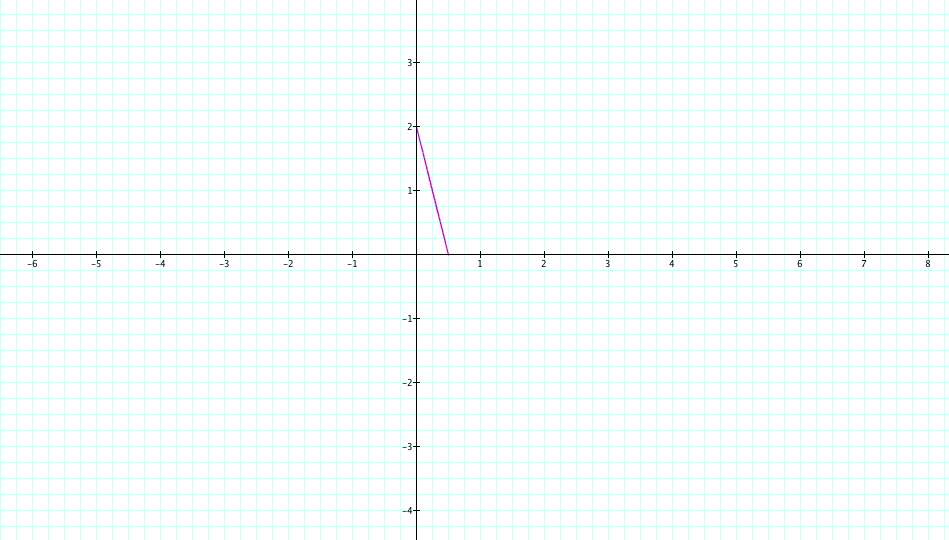
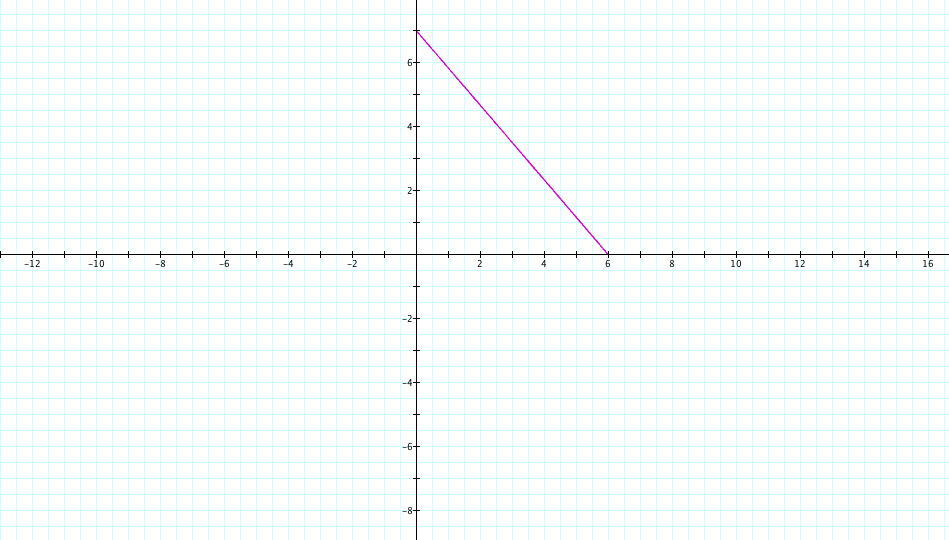
What if a < b? I'm going to assume that the line extends further on the y-axis than the x-axis. The x-intercept is (a, 0) and the y-intercept is (0, b).

a = 0.5 and b = 2	a = 6 and b = 7	a = 11 and b = 17

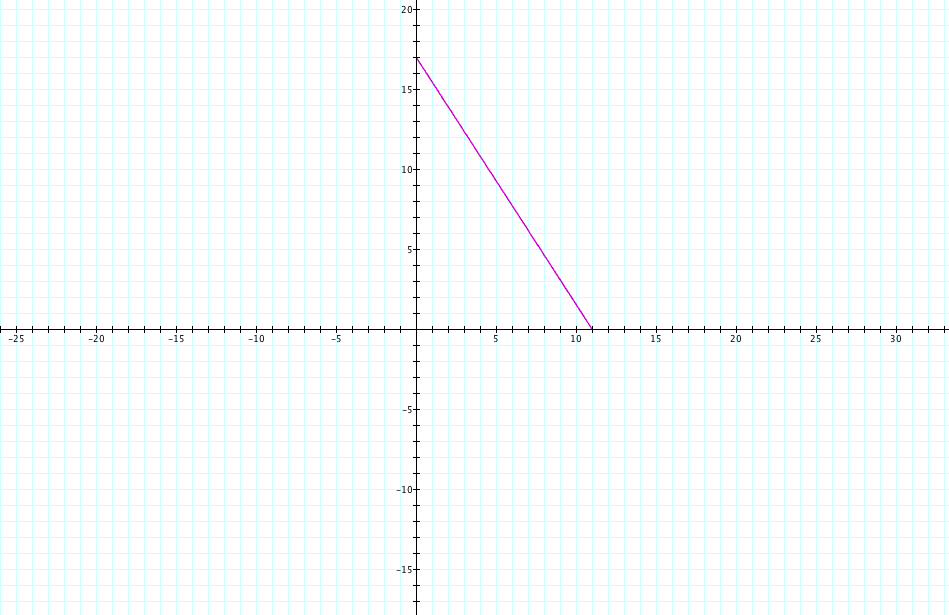Yet again, let me explore what happens when a < b but |a| > |b|.

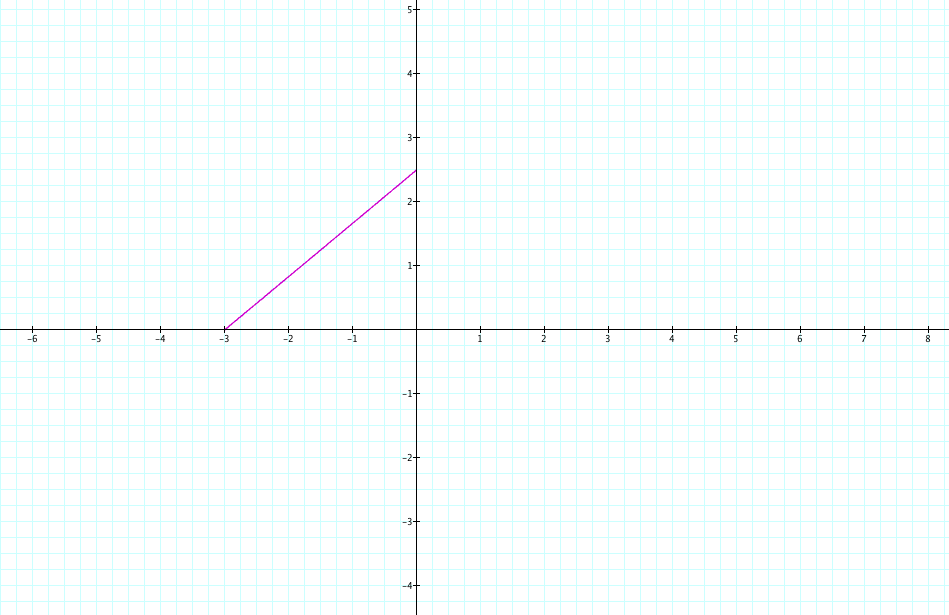a = -3 and b = 2.5

The x-intercept is (-3, 0) and the y-intercept is (0, 2.5).

Let's investigate when you raise the parametric equations to a third degree when .

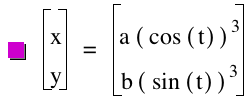where a = b = 1.

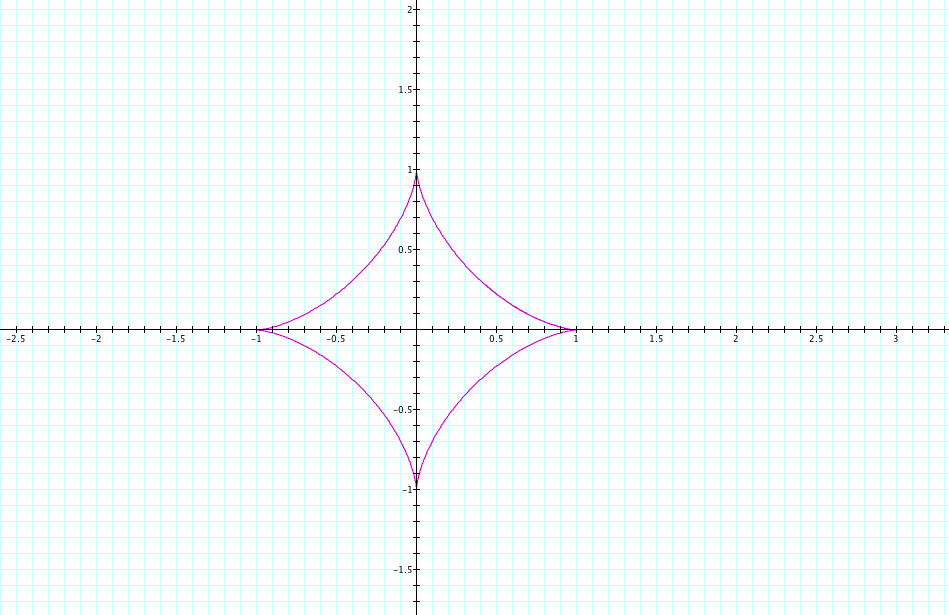It's a very interesting graph. It crosses the same points of x and y intercepts like the parametric equations of the first degree. However, unlike the parametric equations of the first degree, the curves are caved in.

a = b = 8	a = b= 21

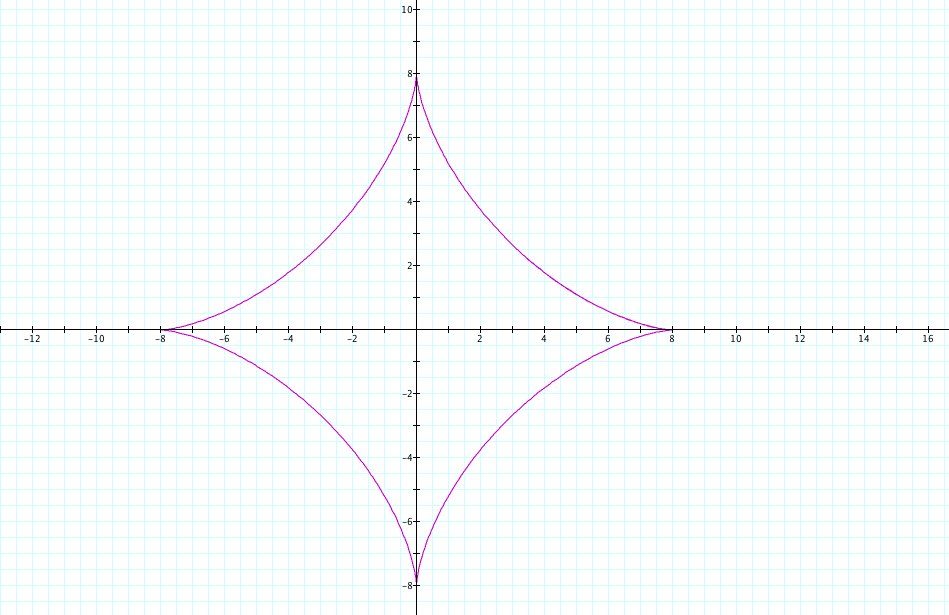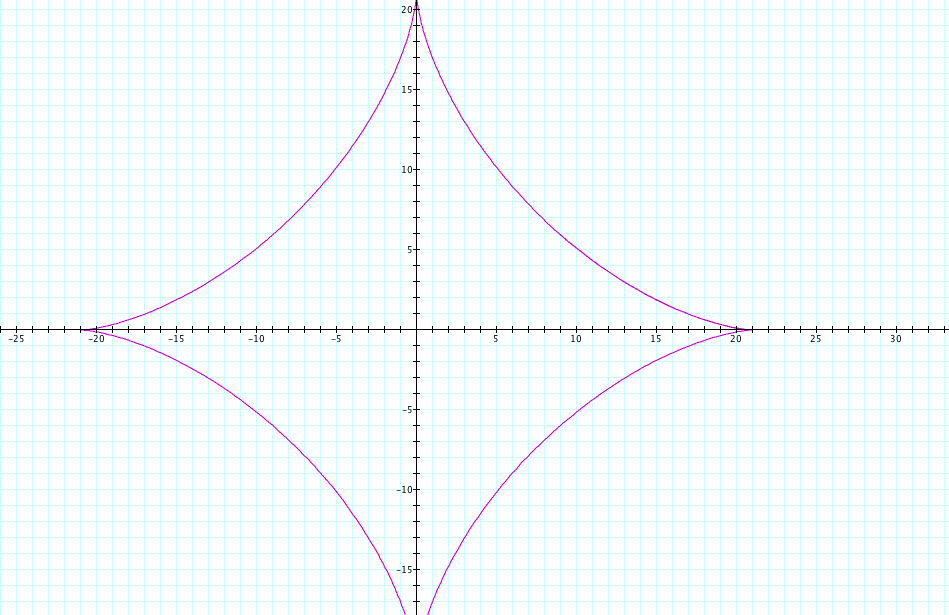Like the parametric equations of the first degree, the sign of a and b doesn't change directions.

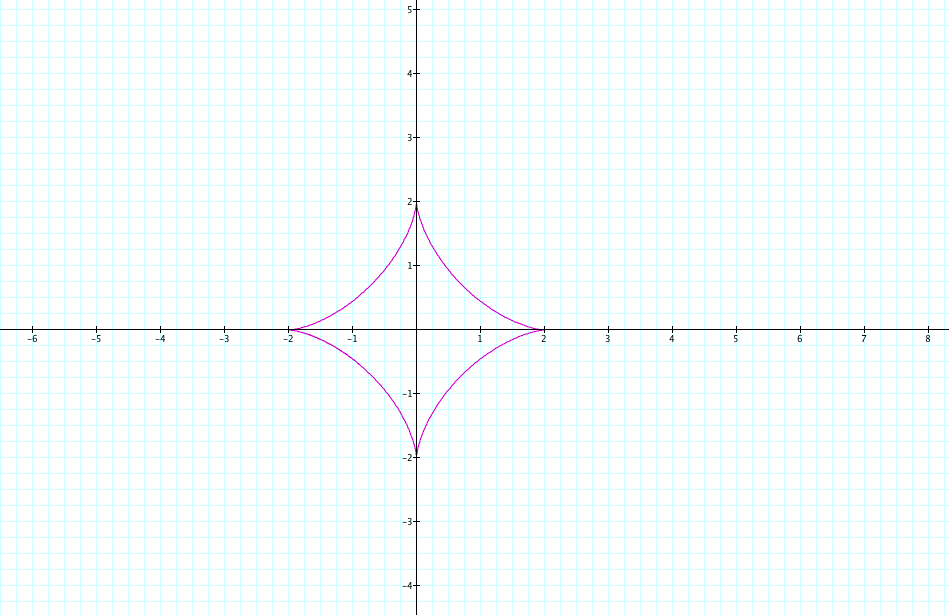When a = b= -2

When a > b.

a = 4 and b = 3.3	a = 5 and b = 1.2	a = -8 and b = -6 (a <b but |a| > |b|)

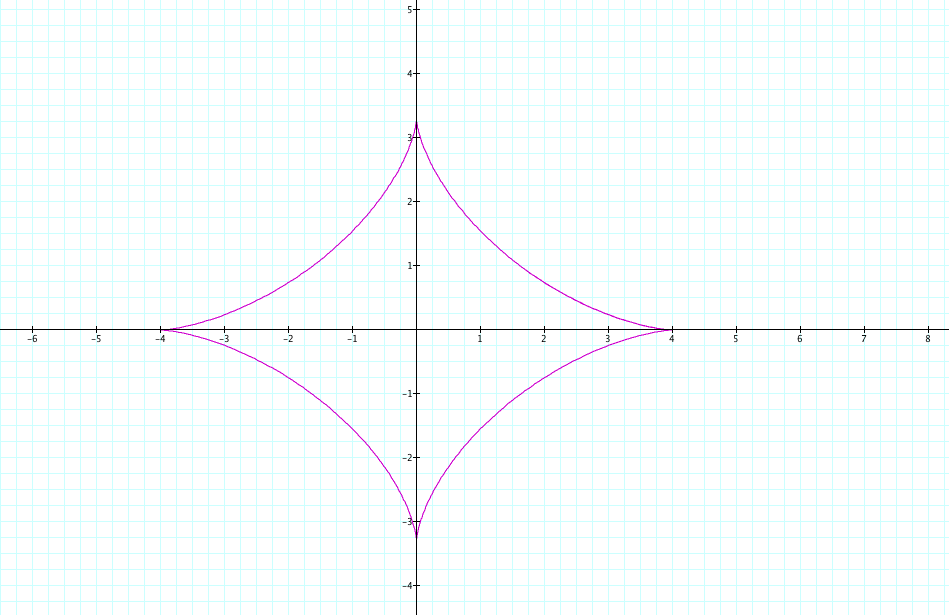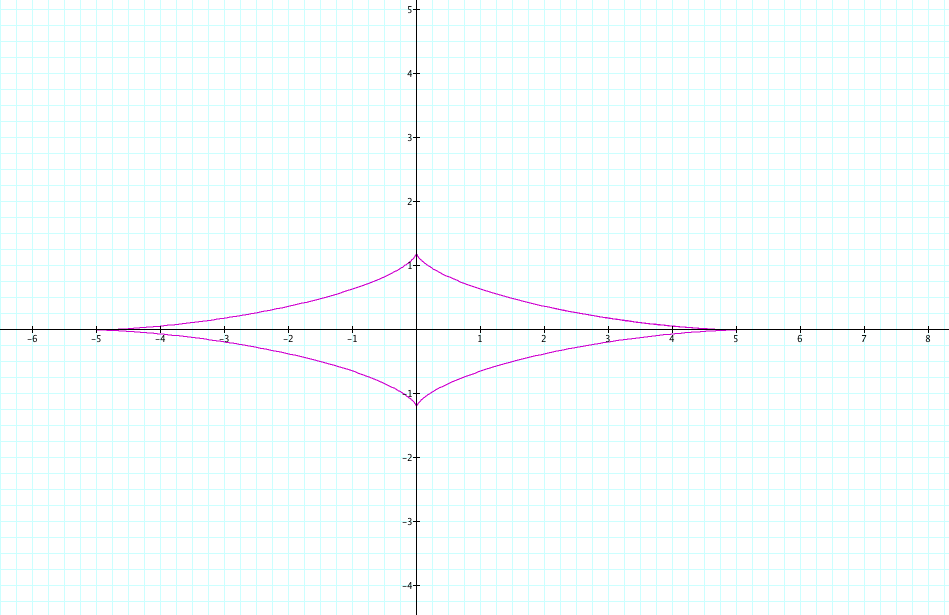x-intercepts are (a, 0) and (-a, 0) and y-intercepts are (0, b) and (0, -b).

When a < b

a = 2 and b= 4.7	a = 3 and b = 7	a = -9 and b = 1 (a < b but |a| > |b|)

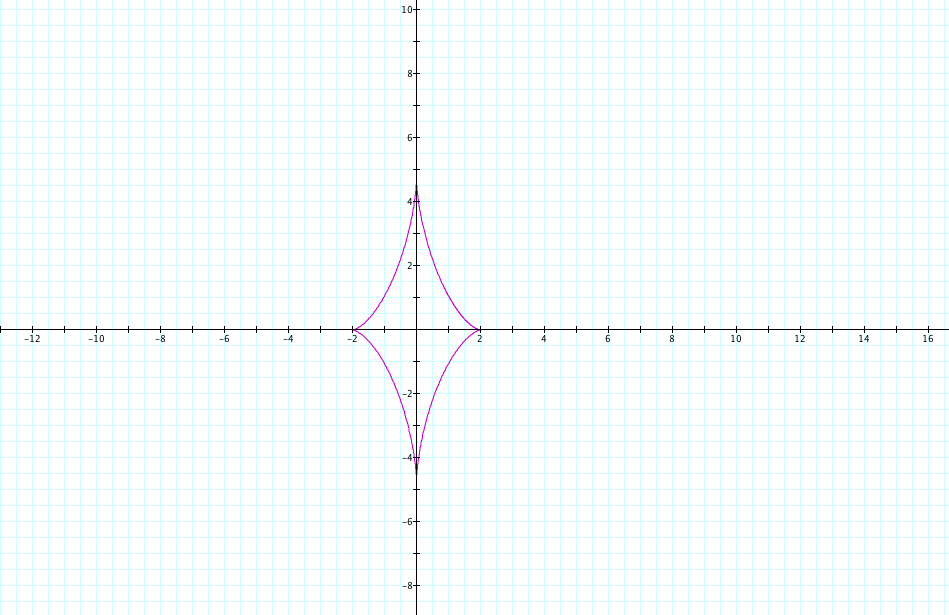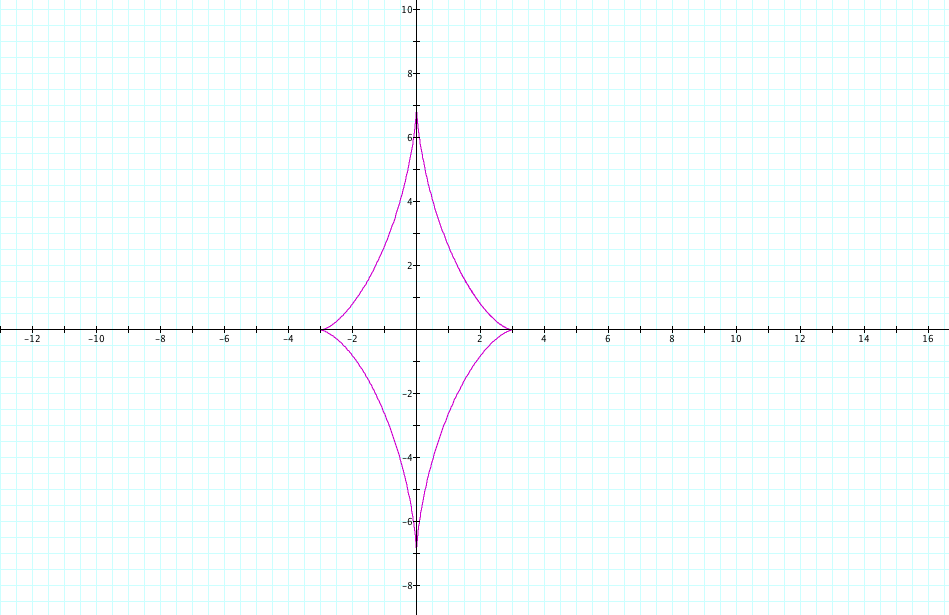As you increase the exponent, the graph looks like... (a = b = 1)

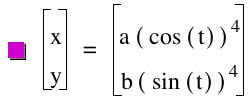
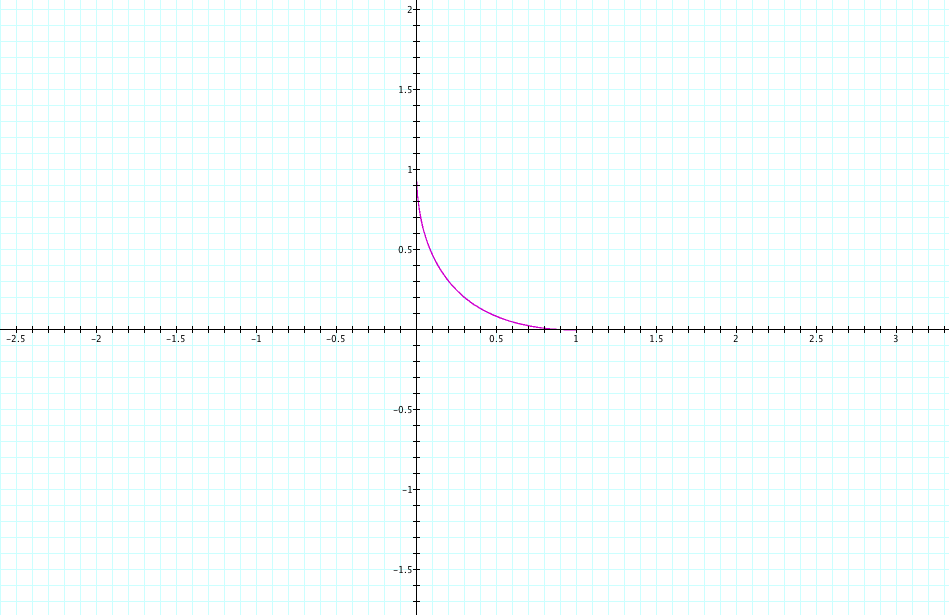
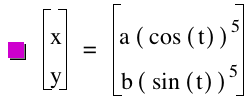
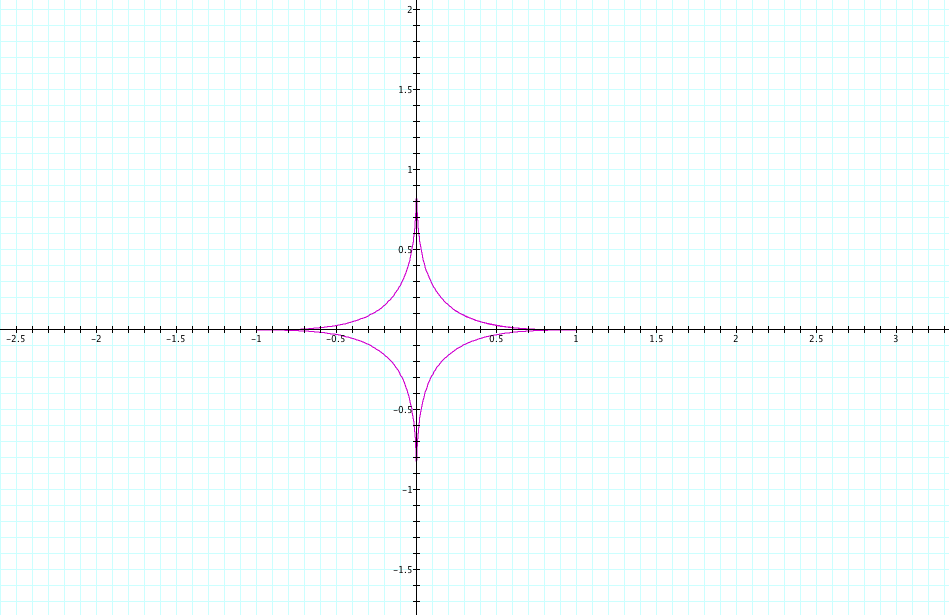
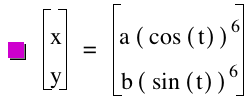
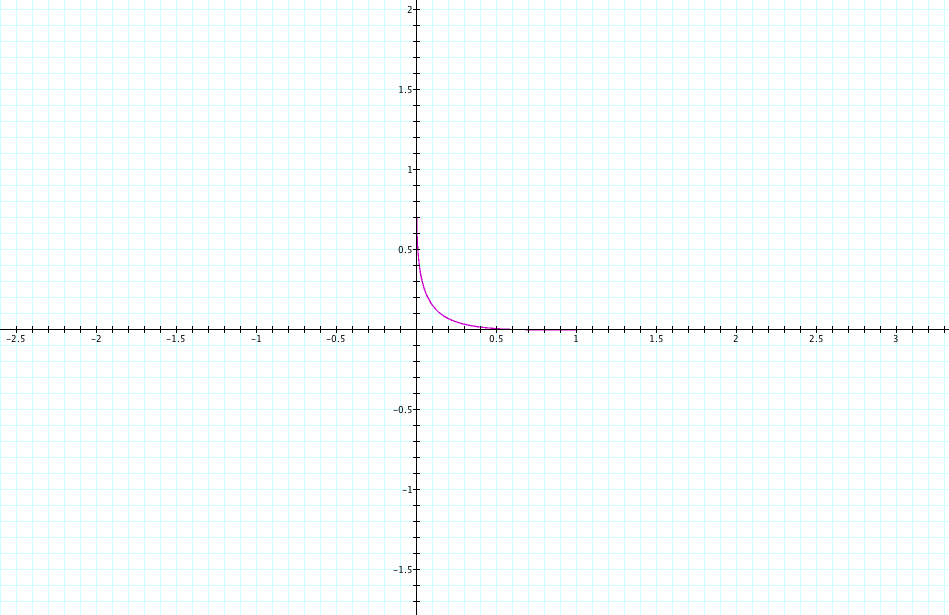
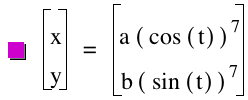
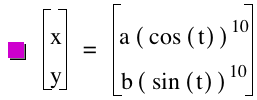
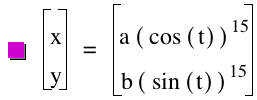
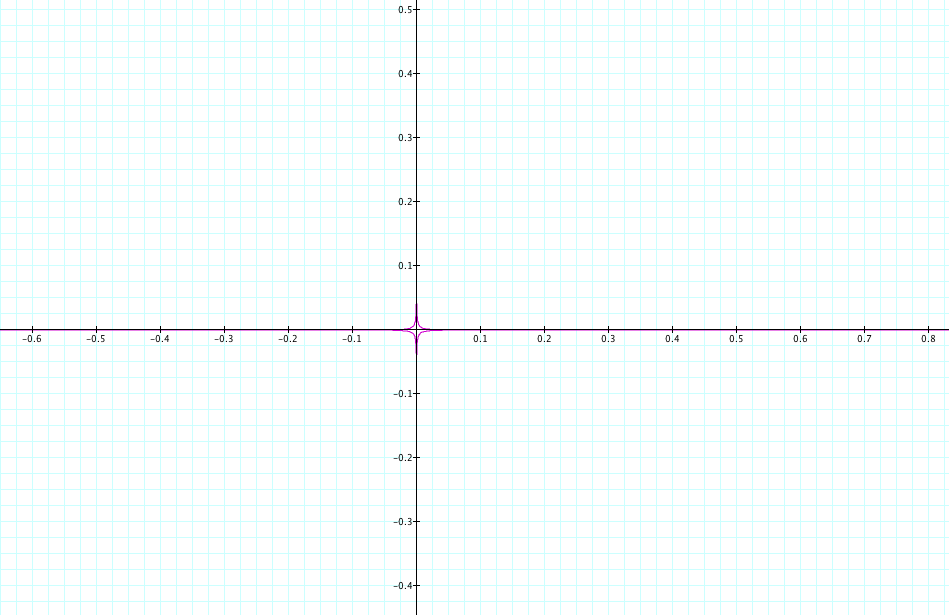
When the degree is even, it looks like a curved line (similar to the parametric equation to the second degree just curvier) that gets closer to the x-axis.

When the degree is odd, it looks like the parametric equation to the third degree that get closer to the origin.

Return to my homepage